Home
This website contains information about polyominoes and their tilings.
Some notions and definitions:
Polyomino - plane geometric figure formed by joining one or more equal squares edge to edge.
For example, figures on the left are polyominoes, but on the right - are not.
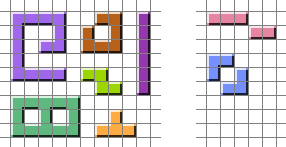
Polyomino tiling - rectangle or other figure filled with translated, rotated and/or reflected copies of one of more different polyominoes without holes and overlapping pieces.
Examples:

You maybe noticed that figure on the right consists of similar twice smaller figures. Such tiling is called 2² reptile (replicating-tile).
Here you can see 3² reptile:

Common multiple - figure that can be filled with copies of two or more polyominoes separately without overlapping pieces.
Example:
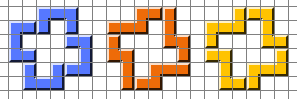
Baiocchi figure - figure with full symmetry of square that can be filled with copies of polyomino without overlapping pieces.
In simple words that is figure which looks like same when rotated at any angle which is multiple of 90 and/or flipped.
Example:
